Doped ceria is a good oxygen ion conductor due to the creation of oxygen vacancies during doping with trivalent oxides e.g. Sm₂O₃. The migration energies of each individual jump are influenced by the interaction of the oxygen vacancy with dopant ions and other oxygen vacancies. The migration energies E_mig are modeled by a combination of a symmetric energy contribution E_sym due to different occupations of the migration edge and a configurational contribution ΔE_con due to the energy change between initial and final state:

The configurational contribution is calculated from pair interactions of the vacancy in the initial and final state:

Structure

The unit cell of the structure is characterized either by direct input of the ‘Lattice Vectors’ or by the ‘Lattice Parameters’, i.e. the lengths and angles of the lattice vectors. The ‘Atoms in the Unit Cell’ are defined by a list of relative positions in the undoped case with corresponding element symbols and charges. The ‚Moving Species’ with the corresponding charge is defined and a list of possible ‘Doping’ atoms can be provided.

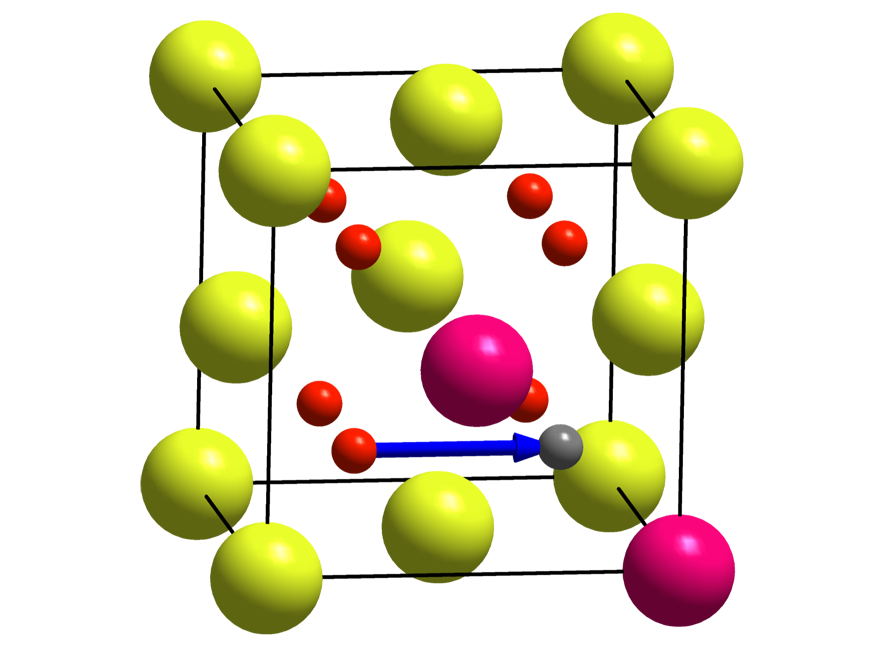
The cubic unit cell of ceria with a lattice parameter of 5.411 Å is defined. The unit cell is composed of 4 Ce⁴⁺ and 8 O^2- ions. The oxygen ions are defined as the mobile species. Sm³⁺ ions are introduced to substitute the Ce⁴⁺ ions. As the introduction of two Sm³⁺ ions leads to the formation of one oxygen vacancy the vacancy to dopant ratio is 0.5. On the right-hand side, the resulting fluorite structure of ceria is presented.

Jumps

In the following section, the maximum distance of the possible jumps is determined by defining a number of ‘Destination’ shells around each mobile ion. The number of shells, which are considered as environment positions, is set around the ‘Start’, ‘Center’ and ‘End’ (or ‘Destination’) position of the migrating vacancy. These settings determine the number of ‘Unique Jumps’ and the number of ‘Environment Positions’ that influence the migration energy. Additional ‘Interaction’ shells can be added to distinguish environment positions with the same distance but different surrounding. For each unique jump, the environment positions can be selected as ‘permuted’, ‘additive’ or ‘constant’.

For the simulation of Sm-doped ceria, only jumps to the nearest neighbor position are considered (‘Destination’). Around ‘Start’ and ‘End’ position, six shells are considered, while around the ‘Center’ position only one shell is considered, corresponding to the migration edge. On the right side, the resulting jump environment is illustrated. An interaction shell of 1 is selected to distinguish between two types of interactions between vacancies in third nearest neighbor position. In the list of the environment positions, the first two positions correspond to the edge cations with a distance of 1.9 Å from the center position. These positions are explicitly permuted while all other interactions are selected as ‘additive’.

Energies

The energies can be modeled independently for each ‘Unique Jump’. Energies are divided into ‘Unique Jump Environments’ that include the list of permuted configurations and into ‘Additive Contributions’.

In the case of Sm-doping, three different edge configurations are possible: Ce-Ce, Ce-Sm, and Sm-Sm. The corresponding energies are entered explicitly and the configurations can be seen on the right-hand side.

All other environment positions contribute to the energy by adding a specific value to the edge energy. This value is by definition zero for Ce⁴⁺ ions and O^2- ions and non-zero for dopant ions and oxygen vacancies. The interactions are illustrated on the right side. Here, the illustration shows the interaction of a position in the oxygen sublattice with the migrating oxygen vacancy jumping from the nearest to the next nearest position.

Job settings

In the job settings, further simulation parameters like the temperature, attempt frequency lattice size, dopant concentration, electric field and number of Monte Carlo steps are entered. A ‘Pre-Run’ can be activated to equilibrate the lattice before recording the ionic conductivity. A ‘Job’ is created from the input data and this job can be duplicated to perform several independent simulations with the same setting. The jobs can be submitted to a local queue or a remote compute cluster. The corresponding settings are entered in the ‘Extended Settings’ on the right-hand side.

Here, simulations are performed at a temperature of 773 K for a lattice of 16x16x16 unit cells with an attempt frequency of 1.47 ⋅ 10¹² Hz. An electric field in x-direction is applied with a strength corresponding to an energy contribution of 0.1 k_BT to the jumps along the field. The distribution of oxygen ions is equilibrated for 100 Monte Carlo steps per oxygen ion and another 100 steps per oxygen ion are performed to obtain the ionic conductivity. In both runs, the abundance of jumps with a certain probability is recorded. The dopant concentration is varied between 1% and 25%.